Hierarchical Dimension
This is a very concise digest of the manuscripts dated by 1984. I'll hardly ever have an opportunity to prepare all the bulk of several hundred pages for publication. Fortunately, there is no need to, as the suggested scheme can be developed in various directions, so that the author's preferences may only be of a personal or historical interest. It would be wiser just to present the general philosophy of dimension, for everybody to make practical conclusions of their own.
|
Since mathematics has forcefully abstracted itself from our everyday experience and restricted itself to entirely formal issues, we can no longer comprehend what it really is, space. Almost anything can be referred to as space nowadays. Finally, mathematicians just abandoned this notion and stack to highly formal constructions with the names containing the word “space” by mere tradition, mainly in the meaning of "manifold". For such abstract objects, a few as abstract definitions of dimension have been introduced, which tell nothing to the heart of a regular person. We are to blindly believe in the stories suggested by the big science, and to be content with following their recipes, pushing the keys in a prescribed order nobody knows why and for which reasons. Given that modern physicists tend to meditate over their formulas rather than take notice of nature, one does not expect any clarifications from that party neither. And, of course, there is no use appealing to science-blinded philosophy for elementary coherence.
Still, there are those who have retained a bit of human curiosity and who sometimes want something palpable, tractable with a kind of intuition about the real thing around us (and the real people dealing with such things) rather than mere combinations of ideograms. Our practical notion of space refers to real world. Yes, life is complex and diverse, and one needs to differently arrange for certain effects; this leads to the thought of numerous spaces, each organized to support some specific activity. In certain cases, such spaces will basically differ by a (quantitative) parameter that we associate with the notion of dimension. However, it is a true notion that we need, that is, a variety of common techniques of constructing spaces of any dimension; don't feed us mere symbolic manipulation.
Let us try to (at least schematically) outline one of the possible solutions. Admitting that any choice requires a lengthy justification, let us, however, start with preliminary hints to the very things to be justified.
In respect to human activity, the idea of space characterizes the available options, the ability of choice. This is how any “spatial” language is used in the everyday life, and life in science does not add any principal difference. So far, the examples from science are more common, as modern philosophy does not accept other authorities. Well, let it be science, with a distant aim of eventually adjusting thus acquired practical experience of space construction to all spheres and levels of activity. Now, let us gradually accumulate the necessary instrumentation.
To make the discussion meaningful, we declare that space does really exist, that it is not a personal freak or sheer fantasy. The world is made that way. And this is how we act in this world. With all that, the existence of space is not of the same kind as the existence of any material things. Space does not exist on itself, without anything at all; space is primarily a relation between things. In philosophy, such matter-dependent existence is called ideal. Conversely, things do not exist regardless of their interrelations, so that any matter assumes some ideality (and this not necessarily space). Under certain conditions, the ideal entities get represented by material things. The word “space” is a mere sound, or pigment on paper, or a bright dot on the screen; as soon as we start practically dealing with space (including theoretical discussions), this things (the word) becomes a conventional designation for space within the current activity or the current topic. Space is objective; still, in every particular case, we approach the idea from one of the possible directions. Any features we discover may refer either to space as it is (its “inner organization” that does not depend on our subjective moods), or to some specific implementation of space in our activity (“realization”). It should be stressed that, in addition to the distinction of the notions of different types (levels), each individual notion develops a layered structure of its own. In this context, we distinguish the natural (“geometrical”) dimension of space and its outer (“topological”) dimension.
0. Point
To be honest, this is not an appropriate idea to start with. Rather, that is what we reach in the conclusion: the summit, the highest degree of abstraction. Still, since this text merely presents something earlier thought up and over, one can afford beginning with the end.
For a constructive theory of dimensionality, a point is the nothing we use to produce anything at all: the vacuum, “zero-dimensional” space, that is, the absence of spatiality as such. The utter impossibility of motion nor action.
As we accept the objectivity of space, a point is the expression of this objectivity. Space contains (or is built of) some points; this is nothing but the affirmation of existence and a specific quality. It is only in respect to its “embracing” space that a point can acquire any definiteness; the point just borrows (inherits) it from its space. That is, there are no points as such: all we have is different spaces that can, in certain contexts, be folded into a point, preserving the same spatial quality.
1. Dimension
In philosophy, there is a category which usually goes under the name of “measure” (not to confuse with the narrow mathematical notion of the same name). The category refers to the very possibility of comparing that to that, when one thing becomes a gauge for another, the unit of measurement. Obviously, what we measure must, in some respect, share the same quality with the chosen unit (that is, be commeasurable with the reference thing). On the other hand, it must differ from the unit, to allow any comparison at all; such distinctions are called quantitative.
Unlike a point, any dimension implies the possibility of motion within certain limits (a “degree of freedom”). So, that is what we call a (one-dimensional) space. For a different choice of the unit, the spatial relations will be expressed by some other numbers (and maybe not numbers at all), which does not influence the objective nature of these relations; the (inner) directedness of the space is as objective, providing a sound basis for the very definition of a single dimension.
Admit that there are several different measures (with the corresponding units of measurement). In this case we speak about a many-dimensional space. In the following we are to discuss the possible interdependencies of the space's dimensions. Here, we observe that, in general, the different dimensions of a space are qualitatively different, and one cannot just add one value to another. For instance (anticipating further discussion), to construct a many-dimensional metric space, one needs to somehow bring the different units to a common measure; in the form for the interval,
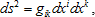
the coefficients g have (physical) dimension
[unit of interval] / [unit i] / [unit k]
Expressing all lengths, say, in meters, we keep in mind a practically available procedure of converting the primary units to the desirable result; in the language, the original units often have different names, such as “a running meter”, “width” (or “breadth”), “height”, and the lots of other names, depending on the kind of what we measure. Consequently, the integrative unit can only be meaningful in the context of an activity requiring that very dimensionality; thus, there is no use to convert all currencies to US dollars where dollars are never introduced in circulation.
In every particular application (a specific activity), we represent a space of a positive integer dimension just listing its dimensions in a definite order. This order may be of practical importance, or may not be. This sequencing does not change the space itself, which implies the entire ensemble of the possible representations, without an absolutely preferable ordering. Still the collection of choices is in no way arbitrary; it is exactly the common basis for all the possible representations of the space that we call its (geometrical) dimension. In other words, dimension is understood as a hierarchy, producing multiple hierarchical structures (the positions of hierarchy). For example, one can observe that the Cartesian product of two spaces with (different) dimensions N1 and N2, is obviously non-commutative, though the overall dimension will equal N1 + N2 in any case. In this model, each many-dimensional space manifests itself as a variety of the decompositions of the total dimension into the sums of partial fragments, which can be graphically pictured as a number of tree-like structures (the possible unfoldings):
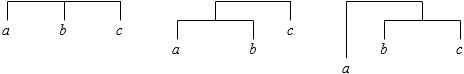 , ,
plus all the permutations in the sequence (a, b, c). In real life, some variants may be practically unfeasible. Thus, to get into an apartment in a city house, we need to first get in, and then make use of an elevator (or a staircase); the inverse order would require the art of climbing the walls.
2. Constraint
The notion of constraint is widely used in analytical mechanics. It can readily be associated with negative dimensions. Indeed, while an additional dimension adds a degree of freedom and increases the total dimensionality of the space, a constraint, conversely, blocks motion along a certain line (not necessarily straight) thus effectively diminishing the dimension of the problem. The simplest constraint must therefore be treated as a space of dimension –1. Any combination of constraints will produce a constraint of a higher rank, producing space of any negative dimension.
The way of imposing a constraint depends on the space where it is to be defined and the choice of parametrization. In particular, when a space is represented by some coordinate system, a constraint can be expressed by an equation somehow combining the coordinates. However, like with the positive dimensions, constraints do not depend on such specific parametrizations. While a dimension conveys the idea of an objective relations between things, a constraint refers to some relation between such relations; this is, so to say, an ideality of a higher level. Still, in many practical cases, when we are primarily occupied with the fundamental contrast of the material and the ideal, rather than the detailed structure of ideality, the distinction between dimensions and constraints is formally irrelevant, and one is free to combine them in any order to produce all kinds of spaces.
Obviously, the spaces of the same overall dimension can be structured in many ways, in accordance with the mode of adding dimensions and imposing constraints. Some combinations may be impossible to practically implement. In an abstract theory, assuming the formal acceptability of all such constructions, the total dimension of a space with constraints is a general characteristic of the possible unfoldings (positions) of hierarchy, hierarchical structures. For example, in atomic physics, a theory of the collective motion of and atomic electron and a hole will make a three-particle problem (accounting for the field of the atomic core); however, the atom is neutral as a whole, and its complex structure will only manifest itself at a closer contact.
3. Projection
Just like constraints, projections effectively diminish the dimension of a space, but they do it in a different manner. A projection relates an N-dimensional space to another space of the dimension Q (the component space), which can be treated as internal space contained in every point of the original space. The dimensions of such inner space are called projections; their dimensionality is evaluated as N / Q. In particular, the components of a one-dimensional space have the dimension of 1 / Q.
This allows constructing spaces of any rational dimension. Imposing constraint on projections rather than the dimensions of the original space, we obtain spaces of negative rational dimension; a projection of an elementary constraint will then have the dimension of –1 / Q. One could readily observe the kinship of negative constraints to the common orthogonalization procedures; thus, projecting a “vector” onto the inner dimensions that are orthogonal to it, we get zero.
A point of the original space can be reconstructed by a complete set of its projections. In the inner space, this means constructing a space of Q dimensions from individual inner dimensions. In terms of outer (“Cartesian”) products, we get the usual equality
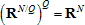
Of course, one is to accurately account for the possible interdependencies, to establish a kind of “orthogonality”, which may only be locally reachable in nonlinear dynamics. This does not change anything in principle.
Real numbers are commonly defined as classes of converging sequences of rational numbers, or the sections of the rational set. Following the same logic, sequences (hierarchical structures) of spaces of rational dimensionality will produce real dimensions. It is important that it is the geometrical (natural) dimension of the space that is real, and not an outer (topological) dimension. In general, no topology is implied by geometry, and geometry does not depend on topology. Some special theories may correlate the procedures of fractal construction to rational-dimensioned spaces, so that topological dimension could follow from geometry, with a kind of conceptual isomorphism. Still, let us stress once again, isomorphism is not equality. For instance, the point of the segment (0, 1) can be neatly mapped to the points of the segment (1, 2), with the entire structure preserved; this in no way means that x = x + 1.
4. Index
Indexing can be understood as the opposite of projection: instead of unfolding an inner space of every point, we attach some outer object to it, thus effectively increasing the overall dimension. This outer thing is used as the “name” of the point, its formal label that can change with the transition from one index system (frame of reference) to another. Such names can be of any nature at all, not necessarily from the mathematical domain. Take, for example physical fields or toponyms. In mathematics, however, indexed spaces are quite common as well. For instance, any coordinate system is of that very kind: we label a spatial point with a cortege of numbers reproducing the chosen sequence of the dimensions of the space. This is an elementary index space of the dimensionality 1, a “vector”. Alternatively, in each point, we can construct a matrix (a tensor of the rank 2) rather than a vector. The components of the tensor we mark with two indexes, so that, if the substrate space has the dimension of N, the components of the tensor will form a space of the dimension N 2. Obviously, indexing with k indexes corresponds to the power k of the dimension of the original (configuration) space.
One might think that the power of a number could be naturally introduced as repeated multiplication:
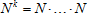 (k times). (k times).
For the square of a space dimension, a similar approach would seemingly give
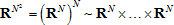 (N times), (N times),
and one could fancy longer chains like that. The problem is that the dots in such expressions do not denote an elementary operation; in fact, this is a sort of “quantifier” which belongs to the next level of logic and hence cannot be defined in terms of the original object area. In fact, we thus mean some activity in the base space. This process may sometimes be programmed, to a certain extent. Much more often, an informal procedure is implied, which makes it an inexhaustible source of ever new mathematical structures. Noting that such repetition, in general, does not need to be limited to an integer count (since we are going to discuss spaces of any real dimension), the above “trivial” definition is utterly unsatisfactory; that is why we accept from the very beginning that exponentiation is an operation of a special kind that cannot, in general, be reduced to multiplication. Still, for a small integer number of indexes, some indexing systems allow establishing a correspondence (isomorphism) between the spaces produced in alternative ways, to preserve the “correspondence principle”.
Dimensional indexing will naturally reproduce the usual properties of the power:

Indeed, if each index may only take a single value, an object with any number of indexes will have a single component; when there is only one index, the number of components equals the dimension of the base space.
Any index lists the dimensions of the base space in a definite order. As mentioned before, this corresponds to unfolding the hierarchy of the space into a specific position. The same hold when the space is being constructed with constraints and projections. The sequence of “constructors” plays the role of a spatial dimension in respect to the index set. Of course, such a space admits index constraints and inner dimensions of the indexes. That is, the number of indexes (and the components of the index space) is generally expressed by a real number. Thus an arbitrary real power of dimension is defined.
The index space of the dimension 0 is readily associated with a scalar field, a numeric function on the base space. Obviously, any dimension in the zeroth power will give zero. For definiteness, let us accept that any power of a zero-dimensional space is to produce an index space with no components. Here, however, there are alternative possibilities: for instance, one might prefer construction of the molds for indexed objects, allowing a definite index structure, but without actual components in the possible positions; such an abstract object does not refer to anything and has nothing to do with the structure of the base space. This is quite like distinguishing complex numbers with zero (or infinite) modulus and a range of phase values (which in not a common choice in the present mathematical theories).
The power (–1) of a dimension N is a constraint of rank N in the index space, which is equivalent to the space with the negative dimension (–N). In the tensor model, such a constraint could be associated with the lower (covariant) index, in contrast to the upper (contravariant) indexes for the positive dimension. Imposing this constraint is simply a convolution of the constraint with one of the upper indexes, so that the total number of indexes will be diminished by one, as one could intuitively expect. However, there are other types of constraint that cannot be directly related to a power of some base space. Thus, merely fixing the value of a single component (or a combination of the components) of a power object, we get a constraint of the dimension –1 on the index space. In general, the values of multiple components get thus interrelated; in respect to the base space, such constraints become symmetries. They do not change the dimensionality of the problem, while significantly influencing dynamics (once again, mind the difference between the geometrical and topological dimension). When there are too many such constraints (above the dimension of the space), symmetries become constraints.
The square of a constraint (a space with the dimension –1), by its sense, is a constraint imposed on a constraint. This effectively corresponds to unfreezing a degree of freedom. That is, for spatial dimensions,
(–1)2 = 1.
5. Branching
Indexing (exponentiation of dimension) is a transition from one level of hierarchy to another level, where the objects are structured unlike the objects (points) of the base space. To produce a regular geometry, we need a special operation enumerating of the components. Of course, such an ordering can be differently achieved. In principle, this does not differ from the enumeration of the dimensions of the base space; however, the necessity of “lifting” the procedure of exponentiation in its result, the transition from many indexes to simple succession (a higher-order index), is always present in the background; this is not a formal trick, but rather a practical act related to the choice of the object area. Given the presence of constraints, such a transition could be compared to canonical transforms in analytical mechanics.
In general,
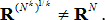
Each instance of exponentiation (albeit to a fractional power) moves us to a higher level of hierarchy, which cannot be unambiguously reduced to a lower level, since there are different unfoldings of the hierarchy, and the same higher level object may result from different hierarchical structures. The parent structures of a power space could be called the branches (folia, replicas) of the base space. These are higher-level objects, which have different dimension but still somehow correspond to each other (up to isomorphism). For example, a two-index space of the dimension 1 can be obtained as a square of either a one-dimensional space, or an elementary constraint; two branches are thus defined, each representing a specific position of the hierarchy of the index space. For index spaces of a more developed structure, the number of branches may increase, and even be infinite.
The branches of an elementary constraint on the index space of the rank 2 (that is, the restrictions on the components of a square matrix) are of a particular interest for the mathematics of dimensionality. Such a constraint has geometrical dimension –1, while the search for the branches means taking a square root. In this way, we come to the notion of imaginary dimension (+i) and imaginary constraint (–i), which can be further expanded into a theory of spaces of any complex dimension, in the simplest case, representable by the product of the real and imaginary parts.
|